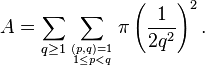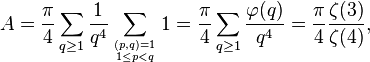Total Area of Ford Circles
There is a link between the area of Ford circles, Euler's totient function φ, the Riemann zeta function ζ, and Apéry's constant ζ(3).
As no two Ford circles intersect, it follows immediately that the total area of the Ford circles
is less than 1. In fact the total area of these Ford circles is given by a convergent sum, which can be evaluated.
From the definition, the area is
Simplifying this expression gives
where the last equality reflects the Dirichlet generating function for Euler's totient function φ(q). Since ζ(4) = π 4/90, this finally becomes
Read more about this topic: Ford Circle
Famous quotes containing the words total, area, ford and/or circles:
“Computers are good at swift, accurate computation and at storing great masses of information. The brain, on the other hand, is not as efficient a number cruncher and its memory is often highly fallible; a basic inexactness is built into its design. The brain’s strong point is its flexibility. It is unsurpassed at making shrewd guesses and at grasping the total meaning of information presented to it.”
—Jeremy Campbell (b. 1931)
“Whether we regard the Women’s Liberation movement as a serious threat, a passing convulsion, or a fashionable idiocy, it is a movement that mounts an attack on practically everything that women value today and introduces the language and sentiments of political confrontation into the area of personal relationships.”
—Arianna Stassinopoulos (b. 1950)
“Obviously, it’s a great privilege and pleasure to be here at the Yale Law School Sesquicentennial Convocation. And I defy anyone to say that and chew gum at the same time.”
—Gerald R. Ford (b. 1913)
“There are some circles in America where it seems to be more socially acceptable to carry a hand-gun than a packet of cigarettes.”
—Katharine Whitehorn (b. 1926)