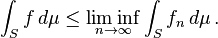Fatou's Lemma - Standard Statement of Fatou's Lemma

Standard Statement of Fatou's Lemma

Let f1, f2, f3, . . . be a sequence of non-negative measurable functions on a measure space (S,Σ,μ). Define the function f : S → a.e. pointwise limit by

f(s) =\liminf_{n\to\infty} f_n(s),\qquad s\in S.

Then f  is measurable and

\int_S f\,d\mu \le \liminf_{n\to\infty} \int_S f_n\,d\mu\,.

Note: The functions are allowed to attain the value +∞ and the integrals may also be infinite.

Read more about this topic:  Fatou's Lemma

Famous quotes containing the words standard and/or statement:

    “... the meanest life, the poorest existence, is attributed to God’s will, but as human beings become more affluent, as their living standard and style begin to ascend the material scale, God descends the scale of responsibility at a commensurate speed.”
    —Maya Angelou (b. 1928)

    “It is commonplace that a problem stated is well on its way to solution, for statement of the nature of a problem signifies that the underlying quality is being transformed into determinate distinctions of terms and relations or has become an object of articulate thought.”
    —John Dewey (1859–1952)