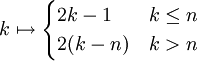Group Theory Aspects
In mathematics, a perfect shuffle can be considered to be an element of the symmetric group.
More generally, in, the perfect shuffle is the permutation that splits the set into 2 piles and interleaves them:
Formally, it sends
Analogously, the -perfect shuffle permutation is the element of that splits the set into k piles and interleaves them.
The -perfect shuffle, denote it, is the composition of the -perfect shuffle with an -cycle, so the sign of is:
The sign is thus 4-periodic:
The first few perfect shuffles are: and are trivial, and is the transposition .
Read more about this topic: Faro Shuffle
Famous quotes containing the words group, theory and/or aspects:
“[The Republicans] offer ... a detailed agenda for national renewal.... [On] reducing illegitimacy ... the state will use ... funds for programs to reduce out-of-wedlock pregnancies, to promote adoption, to establish and operate children’s group homes, to establish and operate residential group homes for unwed mothers, or for any purpose the state deems appropriate. None of the taxpayer funds may be used for abortion services or abortion counseling.”
—Newt Gingrich (b. 1943)
“The theory of truth is a series of truisms.”
—J.L. (John Langshaw)
“Grammar is a tricky, inconsistent thing. Being the backbone of speech and writing, it should, we think, be eminently logical, make perfect sense, like the human skeleton. But, of course, the skeleton is arbitrary, too. Why twelve pairs of ribs rather than eleven or thirteen? Why thirty-two teeth? It has something to do with evolution and functionalism—but only sometimes, not always. So there are aspects of grammar that make good, logical sense, and others that do not.”
—John Simon (b. 1925)