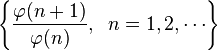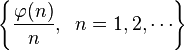Euler's Totient Function - Ratio of Consecutive Values

Ratio of Consecutive Values

In 1950 Somayajulu proved

$\lim\inf \frac{\varphi(n+1)}{\varphi(n)}= 0$ and $\lim\sup \frac{\varphi(n+1)}{\varphi(n)}= \infty.$

In 1954 Schinzel and Sierpiński strengthened this, proving that the set

$\left\{\frac{\varphi(n+1)}{\varphi(n)},\;\;n = 1,2,\cdots\right\}$

is dense in the positive real numbers. They also proved that the set

$\left\{\frac{\varphi(n)}{n},\;\;n = 1,2,\cdots\right\}$

is dense in the interval (0, 1).

Read more about this topic: Euler's Totient Function

Famous quotes containing the words ratio of and/or ratio:

“Personal rights, universally the same, demand a government framed on the ratio of the census: property demands a government framed on the ratio of owners and of owning.”
—Ralph Waldo Emerson (1803–1882)

“A magazine or a newspaper is a shop. Each is an experiment and represents a new focus, a new ratio between commerce and intellect.”
—John Jay Chapman (1862–1933)