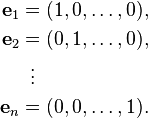Euclidean Space - Real Coordinate Space

Real Coordinate Space

Let R denote the field of real numbers. For any positive integer n, the set of all n-tuples of real numbers forms an n-dimensional vector space over R, which is denoted Rn and sometimes called real coordinate space. An element of Rn is written

where each xi is a real number. The vector space operations on Rn are defined by

The vector space Rn comes with a standard basis:

\begin{align} \mathbf{e}_1 & = (1, 0, \ldots, 0), \\ \mathbf{e}_2 & = (0, 1, \ldots, 0), \\ & {}\,\,\, \vdots \\ \mathbf{e}_n & = (0, 0, \ldots, 1). \end{align}

An arbitrary vector in Rn can then be written in the form

Rn is the prototypical example of a real n-dimensional vector space. In fact, every real n-dimensional vector space V is isomorphic to Rn. This isomorphism is not canonical, however. A choice of isomorphism is equivalent to a choice of basis for V (by looking at the image of the standard basis for Rn in V). The reason for working with arbitrary vector spaces instead of Rn is that it is often preferable to work in a coordinate-free manner (that is, without choosing a preferred basis).

Read more about this topic:  Euclidean Space

Famous quotes containing the words real and/or space:

    “In a real dark night of the soul it is always three o’clock in the morning, day after day.”
    —F. Scott Fitzgerald (1896–1940)

    “In bourgeois society, the French and the industrial revolution transformed the authorization of political space. The political revolution put an end to the formalized hierarchy of the ancien regimé.... Concurrently, the industrial revolution subverted the social hierarchy upon which the old political space was based. It transformed the experience of society from one of vertical hierarchy to one of horizontal class stratification.”
    —Donald M. Lowe, U.S. historian, educator. History of Bourgeois Perception, ch. 4, University of Chicago Press (1982)