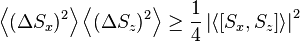EPR Paradox - Mathematical Formulation

Mathematical Formulation

The above discussion can be expressed mathematically using the quantum mechanical formulation of spin. The spin degree of freedom for an electron is associated with a two-dimensional complex Hilbert space H, with each quantum state corresponding to a vector in that space. The operators corresponding to the spin along the x, y, and z direction, denoted Sx, Sy, and Sz respectively, can be represented using the Pauli matrices:

S_x = \frac{\hbar}{2} \begin{bmatrix} 0&1\\1&0\end{bmatrix}, \quad S_y = \frac{\hbar}{2} \begin{bmatrix} 0&-i\\i&0\end{bmatrix}, \quad S_z = \frac{\hbar}{2} \begin{bmatrix} 1&0\\0&-1\end{bmatrix}

where stands for Planck's constant divided by 2π.

The eigenstates of Sz are represented as

\left|+z\right\rang \leftrightarrow \begin{bmatrix}1\\0\end{bmatrix}, \quad \left|-z\right\rang \leftrightarrow \begin{bmatrix}0\\1\end{bmatrix}

and the eigenstates of Sx are represented as

\left|+x\right\rang \leftrightarrow \frac{1}{\sqrt{2}} \begin{bmatrix}1\\1\end{bmatrix}, \quad \left|-x\right\rang \leftrightarrow \frac{1}{\sqrt{2}} \begin{bmatrix}1\\-1\end{bmatrix}

The Hilbert space of the electron pair is, the tensor product of the two electrons' Hilbert spaces. The spin singlet state is

\left|\psi\right\rang = \frac{1}{\sqrt{2}} \bigg (\left|+z\right\rang \otimes \left|-z\right\rang - \left|-z\right\rang \otimes \left|+z\right\rang \bigg)

where the two terms on the right hand side are what we have referred to as state I and state II above.

From the above equations, it can be shown that the spin singlet can also be written as

\left|\psi\right\rang = \frac{-1}{\sqrt{2}} \bigg (\left|+x\right\rang \otimes \left|-x\right\rang - \left|-x\right\rang \otimes \left|+x\right\rang \bigg)

where the terms on the right hand side are what we have referred to as state Ia and state IIa.

To illustrate how this leads to the violation of local realism, we need to show that after Alice's measurement of Sz (or Sx), Bob's value of Sz (or Sx) is uniquely determined, and therefore corresponds to an "element of physical reality". This follows from the principles of measurement in quantum mechanics. When Sz is measured, the system state ψ collapses into an eigenvector of Sz. If the measurement result is +z, this means that immediately after measurement the system state undergoes an orthogonal projection of ψ onto the space of states of the form

For the spin singlet, the new state is

Similarly, if Alice's measurement result is −z, the system undergoes an orthogonal projection onto

which means that the new state is

This implies that the measurement for Sz for Bob's electron is now determined. It will be −z in the first case or +z in the second case.

It remains only to show that Sx and Sz cannot simultaneously possess definite values in quantum mechanics. One may show in a straightforward manner that no possible vector can be an eigenvector of both matrices. More generally, one may use the fact that the operators do not commute,

along with the Heisenberg uncertainty relation

\left\lang (\Delta S_x) ^2 \right\rang \left\lang (\Delta S_z) ^2 \right\rang \ge \frac{1}{4} \left|\left\lang \left \right\rang\right|^2

Read more about this topic:  EPR Paradox

Famous quotes containing the words mathematical and/or formulation:

    “As we speak of poetical beauty, so ought we to speak of mathematical beauty and medical beauty. But we do not do so; and that reason is that we know well what is the object of mathematics, and that it consists in proofs, and what is the object of medicine, and that it consists in healing. But we do not know in what grace consists, which is the object of poetry.”
    —Blaise Pascal (1623–1662)

    “In necessary things, unity; in disputed things, liberty; in all things, charity.”
    —Variously Ascribed.

    The formulation was used as a motto by the English Nonconformist clergyman Richard Baxter (1615-1691)