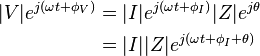Electrical Impedance - Complex Voltage and Current

Complex Voltage and Current

In order to simplify calculations, sinusoidal voltage and current waves are commonly represented as complex-valued functions of time denoted as and .

\begin{align} V &= |V|e^{j(\omega t + \phi_V)} \\ I &= |I|e^{j(\omega t + \phi_I)} \end{align}

Impedance is defined as the ratio of these quantities.

Substituting these into Ohm's law we have

\begin{align} |V| e^{j(\omega t + \phi_V)} &= |I| e^{j(\omega t + \phi_I)} |Z| e^{j\theta} \\ &= |I| |Z| e^{j(\omega t + \phi_I + \theta)} \end{align}

Noting that this must hold for all, we may equate the magnitudes and phases to obtain

\begin{align} |V| &= |I| |Z| \\ \phi_V &= \phi_I + \theta \end{align}

The magnitude equation is the familiar Ohm's law applied to the voltage and current amplitudes, while the second equation defines the phase relationship.

Read more about this topic:  Electrical Impedance

Famous quotes containing the words complex and/or current:

    “We must open our eyes and see that modern civilization has become so complex and the lives of civilized men so interwoven with the lives of other men in other countries as to make it impossible to be in this world and out of it.”
    —Franklin D. Roosevelt (1882–1945)

    “I don’t see America as a mainland, but as a sea, a big ocean. Sometimes a storm arises, a formidable current develops, and it seems it will engulf everything. Wait a moment, another current will appear and bring the first one to naught.”
    —Jacques Maritain (1882–1973)