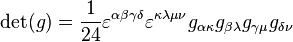Einstein Field Equations - Polynomial Form

Polynomial Form

One might think that EFE are non-polynomial since they contain the inverse of the metric tensor. However, the equations can be arranged so that they contain only the metric tensor and not its inverse. First, the determinant of the metric in 4 dimensions can be written:

\det(g) = \frac{1}{24} \varepsilon^{\alpha\beta\gamma\delta} \varepsilon^{\kappa\lambda\mu\nu} g_{\alpha\kappa} g_{\beta\lambda} g_{\gamma\mu} g_{\delta\nu} \,

using the Levi-Civita symbol; and the inverse of the metric in 4 dimensions can be written as:

g^{\alpha\kappa} = \frac{1}{6} \varepsilon^{\alpha\beta\gamma\delta} \varepsilon^{\kappa\lambda\mu\nu} g_{\beta\lambda} g_{\gamma\mu} g_{\delta\nu} / \det(g) \,.

Substituting this definition of the inverse of the metric into the equations then multiplying both sides by det(g) until there are none left in the denominator results in polynomial equations in the metric tensor and its first and second derivatives. The action from which the equations are derived can also be written in polynomial form by suitable redefinitions of the fields.

Read more about this topic:  Einstein Field Equations

Famous quotes containing the word form:

    “Man is a victim of dope
    In the incurable form of hope.”
    —Ogden Nash (1902–1971)