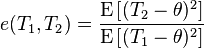Relative Efficiency
If and are estimators for the parameter, then is said to dominate if:
- its mean squared error (MSE) is smaller for at least some value of
- the MSE does not exceed that of for any value of θ.
Formally, dominates if
![\mathrm{E}
\left[ (T_1 - \theta)^2
\right]
\leq
\mathrm{E}
\left[ (T_2-\theta)^2
\right]](http://upload.wikimedia.org/math/3/7/2/372ed8d78dce9eef004a1e6e0c9de3dc.png)
holds for all, with strict inequality holding somewhere.
The relative efficiency is defined as
Although is in general a function of, in many cases the dependence drops out; if this is so, being greater than one would indicate that is preferable, whatever the true value of .
Read more about this topic: Efficient Estimator
Famous quotes containing the words relative and/or efficiency:
“The ungentlemanly expressions and gasconading conduct of yours relative to me yesterday was in true character of yourself and unmask you to the world and plainly show that they were ebullitions of a base mind ... and flow from a source devoid of every refined sentiment or delicate sensations.”
—Andrew Jackson (1767–1845)
““Never hug and kiss your children! Mother love may make your children’s infancy unhappy and prevent them from pursuing a career or getting married!” That’s total hogwash, of course. But it shows on extreme example of what state-of-the-art “scientific” parenting was supposed to be in early twentieth-century America. After all, that was the heyday of efficiency experts, time-and-motion studies, and the like.”
—Lawrence Kutner (20th century)