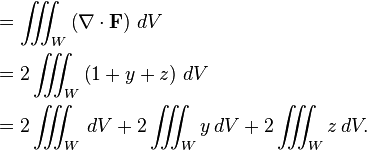Divergence Theorem - Example

Example

Suppose we wish to evaluate

where S is the unit sphere defined by

and F is the vector field

The direct computation of this integral is quite difficult, but we can simplify the derivation of the result using the divergence theorem:

\begin{align} &= \int\!\!\!\!\int\!\!\!\!\int_W\left(\nabla\cdot\mathbf{F}\right) \, dV\\ &= 2\int\!\!\!\!\int\!\!\!\!\int_W\left(1+y+z\right) \, dV\\ &= 2\int\!\!\!\!\int\!\!\!\!\int_W \,dV + 2\int\!\!\!\!\int\!\!\!\!\int_W y \,dV + 2\int\!\!\!\!\int\!\!\!\!\int_W z \,dV. \end{align}

where W is the unit ball (i.e., the interior of the unit sphere, ). Since the function is positive in one hemisphere of W and negative in the other, in an equal and opposite way, its total integral over W is zero. The same is true for :

Therefore,

because the unit ball W has volume

Read more about this topic:  Divergence Theorem

Famous quotes containing the word example:

    “Our intellect is not the most subtle, the most powerful, the most appropriate, instrument for revealing the truth. It is life that, little by little, example by example, permits us to see that what is most important to our heart, or to our mind, is learned not by reasoning but through other agencies. Then it is that the intellect, observing their superiority, abdicates its control to them upon reasoned grounds and agrees to become their collaborator and lackey.”
    —Marcel Proust (1871–1922)