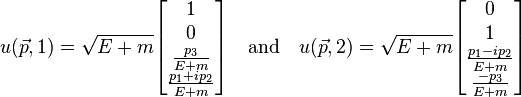Dirac Spinor - Four-spinor For Particles

Four-spinor For Particles

Particles are defined as having positive energy. The normalization for the four-spinor ω is chosen so that . These spinors are denoted as u:

u(\vec{p}, s) = \sqrt{E+m} \begin{bmatrix} \phi^{(s)}\\ \frac{\vec{\sigma} \cdot \vec{p} }{E+m} \phi^{(s)} \end{bmatrix} \,

where s = 1 or 2 (spin "up" or "down")

Explicitly,

u(\vec{p}, 1) = \sqrt{E+m} \begin{bmatrix} 1\\ 0\\ \frac{p_3}{E+m} \\ \frac{p_1 + i p_2}{E+m} \end{bmatrix} \quad \mathrm{and} \quad u(\vec{p}, 2) = \sqrt{E+m} \begin{bmatrix} 0\\ 1\\ \frac{p_1 - i p_2}{E+m} \\ \frac{-p_3}{E+m} \end{bmatrix}

Read more about this topic:  Dirac Spinor

Famous quotes containing the word particles:

    “O my countrymen!—be nice;Mbe cautious of your language;—and never, O! never let it be forgotten upon what small particles your eloquence and your fame depend.”
    —Laurence Sterne (1713–1768)