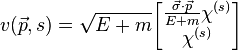Dirac Spinor - Four-spinor For Anti-particles

Four-spinor For Anti-particles

Anti-particles having positive energy are defined as particles having negative energy and propagating backward in time. Hence changing the sign of and in the four-spinor for particles will give the four-spinor for anti-particles:

$v(\vec{p},s) = \sqrt{E+m} \begin{bmatrix} \frac{\vec{\sigma} \cdot \vec{p} }{E+m} \chi^{(s)}\\ \chi^{(s)} \end{bmatrix} \,$

Here we choose the solutions. Explicitly,

$v(\vec{p}, 1) = \sqrt{E+m} \begin{bmatrix} \frac{p_1 - i p_2}{E+m} \\ \frac{-p_3}{E+m} \\ 0\\ 1 \end{bmatrix} \quad \mathrm{and} \quad v(\vec{p}, 2) = \sqrt{E+m} \begin{bmatrix} \frac{p_3}{E+m} \\ \frac{p_1 + i p_2}{E+m} \\ 1\\ 0\\ \end{bmatrix}$

Read more about this topic: Dirac Spinor