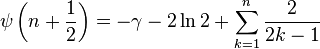Digamma Function - Relation To Harmonic Numbers

Relation To Harmonic Numbers

The digamma function, often denoted also as ψ0(x), ψ0(x) or (after the shape of the archaic Greek letter Ϝ digamma), is related to the harmonic numbers in that

where Hn is the n harmonic number, and γ is the Euler-Mascheroni constant. For half-integer values, it may be expressed as

\psi\left(n+{\frac{1}{2}}\right) = -\gamma - 2\ln 2 + \sum_{k=1}^n \frac{2}{2k-1}

Read more about this topic:  Digamma Function

Famous quotes containing the words relation to, relation, harmonic and/or numbers:

    “... a worker was seldom so much annoyed by what he got as by what he got in relation to his fellow workers.”
    —Mary Barnett Gilson (1877–?)

    “Whoever has a keen eye for profits, is blind in relation to his craft.”
    —Sophocles (497–406/5 B.C.)

    “For decades child development experts have erroneously directed parents to sing with one voice, a unison chorus of values, politics, disciplinary and loving styles. But duets have greater harmonic possibilities and are more interesting to listen to, so long as cacophony or dissonance remains at acceptable levels.”
    —Kyle D. Pruett (20th century)

    “The forward Youth that would appear
    Must now forsake his Muses dear,
    Nor in the Shadows sing
    His Numbers languishing.”
    —Andrew Marvell (1621–1678)