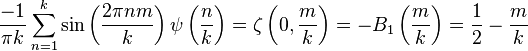Digamma Function - Gaussian Sum

Gaussian Sum

The digamma has a Gaussian sum of the form

\frac{-1}{\pi k} \sum_{n=1}^k \sin \left( \frac{2\pi nm}{k}\right) \psi \left(\frac{n}{k}\right) = \zeta\left(0,\frac{m}{k}\right) = -B_1 \left(\frac{m}{k}\right) = \frac{1}{2} - \frac{m}{k}

for integers . Here, ΞΆ(s,q) is the Hurwitz zeta function and is a Bernoulli polynomial. A special case of the multiplication theorem is

\sum_{n=1}^k \psi \left(\frac{n}{k}\right) =-k(\gamma+\log k),

and a neat generalization of this is

where q must be a natural number, but 1-qa not.

Read more about this topic:  Digamma Function

Famous quotes containing the word sum:

    “Never is a historic deed already completed when it is done but always only when it is handed down to posterity. What we call “history” by no means represents the sum total of all significant deeds.... World history ... only comprises that tiny lighted sector which chanced to be placed in the spotlight by poetic or scholarly depictions.”
    —Stefan Zweig (18811942)