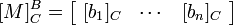Basis Transformation Matrix
Let B and C be two different bases of a vector space V, and let us mark with the matrix which has columns consisting of the C representation of basis vectors b1, b2, ..., bn:
This matrix is referred to as the basis transformation matrix from B to C, and can be used for transforming any vector v from a B representation to a C representation, according to the following theorem:
If E is the standard basis, the transformation from B to E can be represented with the following simplified notation:
where
- and
Read more about this topic: Coordinate Vector
Famous quotes containing the words basis and/or matrix:
“Our fathers and grandfathers who poured over the Midwest were self-reliant, rugged, God-fearing people of indomitable courage.... They asked only for freedom of opportunity and equal chance. In these conceptions lies the real basis of American democracy. They and their fathers give a genius to American institutions that distinguished our people from any other in the world.”
—Herbert Hoover (1874–1964)
““The matrix is God?”
“In a manner of speaking, although it would be more accurate ... to say that the matrix has a God, since this being’s omniscience and omnipotence are assumed to be limited to the matrix.”
“If it has limits, it isn’t omnipotent.”
“Exactly.... Cyberspace exists, insofar as it can be said to exist, by virtue of human agency.””
—William Gibson (b. 1948)