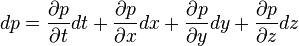Control Volume - Substantive Derivative

Substantive Derivative

Computations in fluid mechanics often require that the regular time derivation operator is replaced by the substantive derivative operator . This can be seen as follows.

Consider a bug that is moving through a volume where there is some scalar, e.g. pressure, that varies with time and position: .

If the bug during the time interval from to moves from to then the bug experiences a change in the scalar value,

dp = \frac{\partial p}{\partial t}dt + \frac{\partial p}{\partial x}dx + \frac{\partial p}{\partial y}dy + \frac{\partial p}{\partial z}dz

(the total differential). If the bug is moving with velocity the change in position is and we may write

\begin{alignat}{2} dp & = \frac{\partial p}{\partial t}dt + \frac{\partial p}{\partial x}v_xdt + \frac{\partial p}{\partial y}v_ydt + \frac{\partial p}{\partial z}v_zdt \\ & = \left( \frac{\partial p}{\partial t} + \frac{\partial p}{\partial x}v_x + \frac{\partial p}{\partial y}v_y + \frac{\partial p}{\partial z}v_z \right)dt \\ & = \left( \frac{\partial p}{\partial t} + \mathbf v\cdot\nabla p \right)dt. \\ \end{alignat}

where is the gradient of the scalar field p. If the bug is just a fluid particle moving with the fluid's velocity field, the same formula applies, but now the velocity vector is that of the fluid. The last parenthesized expression is the substantive derivative of the scalar pressure. Since the pressure p in this computation is an arbitrary scalar field, we may abstract it and write the substantive derivative operator as

Read more about this topic:  Control Volume

Famous quotes containing the word derivative:

    “Poor John Field!—I trust he does not read this, unless he will improve by it,—thinking to live by some derivative old-country mode in this primitive new country.... With his horizon all his own, yet he a poor man, born to be poor, with his inherited Irish poverty or poor life, his Adam’s grandmother and boggy ways, not to rise in this world, he nor his posterity, till their wading webbed bog-trotting feet get talaria to their heels.”
    —Henry David Thoreau (1817–1862)