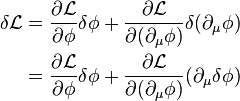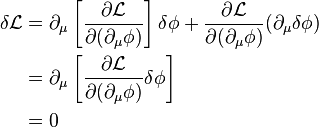Continuity Equation - Conserved Currents From Noether's Theorem

Conserved Currents From Noether's Theorem

For more detailed explanations and derivations, see Noether's theorem.

If a field φ(r, t), which can be a tensor field of any order describing (say) gravitational, electromagnetic, or even quantum fields, is varied by a small amount;

then Noether's theorem states the Lagrangian L (also Lagrangian density ℒ for fields) is invariant (doesn't change) under a continuous symmetry (the field is a continuous variable):

This leads to conserved current densities in a completely general form:

because they satisfy the continuity equation

Derivation of conserved currents from Lagrangian density

The variation in is:

\begin{align} \delta\mathcal{L} & = \frac{\partial \mathcal{L}}{\partial \phi}\delta\phi + \frac{\partial \mathcal{L}}{\partial (\partial_\mu \phi)} \delta (\partial_\mu \phi)\\ & = \frac{\partial \mathcal{L}}{\partial \phi}\delta\phi + \frac{\partial \mathcal{L}}{\partial (\partial_\mu \phi)} (\partial_\mu \delta \phi)\\ \end{align}

next using the Euler-Lagrange equations for a field:

the variation is:

\begin{align} \delta\mathcal{L} & = \partial_\mu\left\delta\phi + \frac{\partial \mathcal{L}}{\partial (\partial_\mu \phi)} (\partial_\mu \delta \phi)\\ & = \partial_\mu\left \\ & = 0 \end{align}

where the product rule has been used, so we obtain the continuity equation in a different general form:

So the conserved current (density) has to be:

Integrating Jμ over a spacelike region of volume gives the total amount of conserved quantity within that volume:

Read more about this topic:  Continuity Equation

Famous quotes containing the words currents and/or theorem:

    “Standing on the bare ground,—my head bathed by the blithe air, and uplifted into infinite space,—all mean egotism vanishes. I become a transparent eye-ball; I am nothing; I see all; the currents of the Universal Being circulate through me; I am part and particle of God.”
    —Ralph Waldo Emerson (1803–1882)

    “To insure the adoration of a theorem for any length of time, faith is not enough, a police force is needed as well.”
    —Albert Camus (1913–1960)