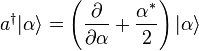Coherent States - Mathematical Characteristics of The Canonical Coherent States

Mathematical Characteristics of The Canonical Coherent States

The canonical coherent states described so far have three properties that are mutually equivalent, since each of them completely specifies the state, namely,

  1. They are eigenvectors of the annihilation operator: .
  2. They are obtained from the vacuum by application of a unitary displacement operator: .
  3. They are states of (balanced) minimal uncertainty: .

Each of these properties may lead to generalizations, in general different from each other (see the article 'Coherent states in mathematical physics' for some of these). We emphasize that coherent states have mathematical features that are very different from those of a Fock state; for instance two different coherent states are not orthogonal:

(this is related to the fact that they are eigenvectors of the non-self-adjoint operator ).

Thus, if the oscillator is in the quantum state it is also with nonzero probability in the other quantum state (but the farther apart the states are situated in phase space, the lower the probability is). However, since they obey a closure relation, any state can be decomposed on the set of coherent states. They hence form an overcomplete basis in which one can diagonally decompose any state. This is the premise for the Sudarshan-Glauber P representation. This closure relation can be expressed by the resolution of the identity operator in the vector space of quantum states:

\frac{1}{\pi} \int |\alpha\rangle\langle\alpha| d^2\alpha = I \qquad d^2\alpha \equiv d\Re(\alpha) \, d\Im(\alpha).

Another difficulty is that has no eigenket (and has no eigenbra). The following formal equality is the closest substitute and turns out to be very useful for technical computations:

a^{\dagger}|\alpha\rangle=\left({\partial\over\partial\alpha}+{\alpha^*\over 2}\right)|\alpha\rangle

The last state is known as Agarwal state or photon-added coherent state and denoted as Normalized Agarwal states for order can be expressed as

The resolution of the identity may be derived (restricting to one spatial dimension for simplicity) by taking matrix elements between eigenstates of position, on both sides of the equation. On the right-hand side, this immediately gives . On the left-hand side, the same is obtained by inserting

\psi^\alpha(x,t) = \langle x | \alpha(t)\rangle

from the previous section (time is arbitrary), then integrating over using the Fourier representation of the delta function, and then performing a Gaussian integral over .

The resolution of the identity may also be expressed in terms of particle position and momentum. For each coordinate dimension, using an adapted notation with new meaning of ,

|\alpha\rangle \equiv |x,p\rangle \qquad \qquad x \equiv \langle \hat{x} \rangle \qquad\qquad p \equiv \langle \hat{p} \rangle

the closure relation of coherent states reads

I = \int |x,p\rangle \, \langle x,p| ~ \frac{\mathrm{d}x\,\mathrm{d}p}{2\pi\hbar}

This can be inserted in any quantum-mechanical expectation value, relating it to some quasi-classical phase-space integral and explaining, in particular, the origin of normalisation factors for classical partition functions consistent with quantum mechanics.

In addition to being an exact eigenstate of annihilation operators, a coherent state is an approximate common eigenstate of particle position and momentum. Restricting to one dimension again,

\hat{x} |x,p\rangle \approx x |x,p\rangle \qquad \qquad \hat{p} |x,p\rangle \approx p |x,p\rangle

The error in these approximations is measured by the uncertainties of position and momentum,

\langle x, p | \left(\hat{x} - x \right)^2 |x,p\rangle = \left(\Delta x\right)^2 \qquad \qquad \langle x, p | \left(\hat{p} - p \right)^2 |x,p\rangle = \left(\Delta p\right)^2

Read more about this topic:  Coherent States

Famous quotes containing the words mathematical, canonical, coherent and/or states:

    “It is by a mathematical point only that we are wise, as the sailor or the fugitive slave keeps the polestar in his eye; but that is sufficient guidance for all our life. We may not arrive at our port within a calculable period, but we would preserve the true course.”
    —Henry David Thoreau (1817–1862)

    “If God bestowed immortality on every man then when he made him, and he made many to whom he never purposed to give his saving grace, what did his Lordship think that God gave any man immortality with purpose only to make him capable of immortal torments? It is a hard saying, and I think cannot piously be believed. I am sure it can never be proved by the canonical Scripture.”
    —Thomas Hobbes (1579–1688)

    “We have good reason to believe that memories of early childhood do not persist in consciousness because of the absence or fragmentary character of language covering this period. Words serve as fixatives for mental images. . . . Even at the end of the second year of life when word tags exist for a number of objects in the child’s life, these words are discrete and do not yet bind together the parts of an experience or organize them in a way that can produce a coherent memory.”
    —Selma H. Fraiberg (20th century)

    “Colonel [John Charles] Fremont. Not a good picture, but will do to indicate my politics this year. For free States and against new slave States.”
    —Rutherford Birchard Hayes (1822–1893)