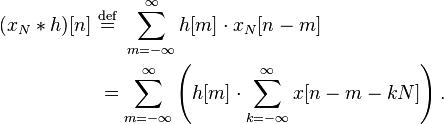Circular Convolution - Discrete Sequences

Discrete Sequences

Similarly, for discrete sequences and period N, we can write the circular convolution of functions h and x as:

\begin{align} (x_N * h) \ &\stackrel{\mathrm{def}}{=} \ \sum_{m=-\infty}^\infty h \cdot x_N \\ &= \sum_{m=-\infty}^\infty \left( h \cdot \sum_{k=-\infty}^\infty x \right). \end{align}

This corresponds to matrix multiplication, and the kernel of the integral transform is a circulant matrix.

Read more about this topic:  Circular Convolution

Famous quotes containing the word discrete:

    “We have good reason to believe that memories of early childhood do not persist in consciousness because of the absence or fragmentary character of language covering this period. Words serve as fixatives for mental images. . . . Even at the end of the second year of life when word tags exist for a number of objects in the child’s life, these words are discrete and do not yet bind together the parts of an experience or organize them in a way that can produce a coherent memory.”
    —Selma H. Fraiberg (20th century)