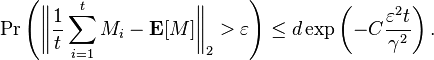Matrix Chernoff Bound
Rudolf Ahlswede and Andreas Winter introduced (Ahlswede & Winter 2003) a Chernoff bound for matrix-valued random variables.
If is distributed according to some distribution over matrices with zero mean, and if are independent copies of then for any ,
where holds almost surely and is an absolute constant.
Notice that the number of samples in the inequality depends logarithmically on . In general, unfortunately, such a dependency is inevitable: take for example a diagonal random sign matrix of dimension . The operator norm of the sum of independent samples is precisely the maximum deviation among independent random walks of length . In order to achieve a fixed bound on the maximum deviation with constant probability, it is easy to see that should grow logarithmically with in this scenario.
The following theorem can be obtained by assuming has low rank, in order to avoid the dependency on the dimensions.
Read more about this topic: Chernoff Bound
Famous quotes containing the words matrix and/or bound:
“In all cultures, the family imprints its members with selfhood. Human experience of identity has two elements; a sense of belonging and a sense of being separate. The laboratory in which these ingredients are mixed and dispensed is the family, the matrix of identity.”
—Salvador Minuchin (20th century)
“The essence of the modern state is that the universal be bound up with the complete freedom of its particular members and with private well-being, that thus the interests of family and civil society must concentrate themselves on the state.... It is only when both these moments subsist in their strength that the state can be regarded as articulated and genuinely organized.”
—Georg Wilhelm Friedrich Hegel (1770–1831)