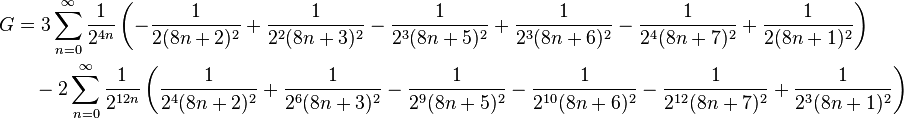Catalan's Constant - Quickly Converging Series

Quickly Converging Series

The following two formulas involve quickly converging series, and are thus appropriate for numerical computation:

\begin{align} G & = 3 \sum_{n=0}^\infty \frac{1}{2^{4n}} \left( -\frac{1}{2(8n+2)^2} +\frac{1}{2^2(8n+3)^2} -\frac{1}{2^3(8n+5)^2} +\frac{1}{2^3(8n+6)^2} -\frac{1}{2^4(8n+7)^2} +\frac{1}{2(8n+1)^2} \right) \\ & {}\quad -2 \sum_{n=0}^\infty \frac{1}{2^{12n}} \left( \frac{1}{2^4(8n+2)^2} +\frac{1}{2^6(8n+3)^2} -\frac{1}{2^9(8n+5)^2} -\frac{1}{2^{10} (8n+6)^2} -\frac{1}{2^{12} (8n+7)^2} +\frac{1}{2^3(8n+1)^2} \right) \end{align}

and

The theoretical foundations for such series is given by Broadhurst (the first formula) and Ramanujan (the second formula). The algorithms for fast evaluation of the Catalan constant is constructed by E. Karatsuba.

Read more about this topic:  Catalan's Constant

Famous quotes containing the words quickly, converging and/or series:

    “But O, young beauty of the woods,
    Whom Nature courts with fruits and flowers,
    Gather the flowers, but spare the buds;
    Lest Flora, angry at thy crime
    To kill her infants in their prime,
    Do quickly make the example yours;
    And ere we see,
    Nip in the blossom all our hopes and thee.”
    —Andrew Marvell (1621–1678)

    “It might become a wheel spoked red and white
    In alternate stripes converging at a point
    Of flame on the line, with a second wheel below,
    Just rising, accompanying, arranged to cross,
    Through weltering illuminations, humps
    Of billows, downward, toward the drift-fire shore.”
    —Wallace Stevens (1879–1955)

    “Depression moods lead, almost invariably, to accidents. But, when they occur, our mood changes again, since the accident shows we can draw the world in our wake, and that we still retain some degree of power even when our spirits are low. A series of accidents creates a positively light-hearted state, out of consideration for this strange power.”
    —Jean Baudrillard (b. 1929)