Cat State - in Quantum Optics

In Quantum Optics

In quantum optics, a cat state is defined as the coherent superposition of two coherent states with opposite phase:

|\mathrm{cat}_e\rangle \propto|\alpha\rangle+|{-}\alpha\rangle ,

where

|\alpha\rangle =e^{-{|\alpha|^2\over2}}\sum_{n=0}^{\infty}{\alpha^n\over\sqrt{n!}}|n\rangle ,

and

|{-}\alpha\rangle =e^{-{|{-}\alpha|^2\over2}}\sum_{n=0}^{\infty}{({-}\alpha)^n\over\sqrt{n!}}|n\rangle ,

are coherent states defined in the number (Fock) basis. Notice that if we add the two states together, the resulting cat state only contains even Fock state terms

|\mathrm{cat}_e\rangle \propto 2e^{-{|\alpha|^2\over2}}\left({\alpha^0\over\sqrt{0!}}|0\rangle+{\alpha^2\over\sqrt{2!}}|2\rangle+{\alpha^4\over\sqrt{4!}}|4\rangle+\dots\right) .

As a result of this property, the above cat state is often refereed to as an even cat state. Alternatively, we can define an odd cat state as

|\mathrm{cat}_o\rangle \propto|\alpha\rangle-|{-}\alpha\rangle ,

which only contains odd Fock states

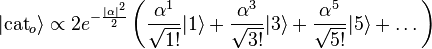|\mathrm{cat}_o\rangle \propto 2e^{-{|\alpha|^2\over2}}\left({\alpha^1\over\sqrt{1!}}|1\rangle+{\alpha^3\over\sqrt{3!}}|3\rangle+{\alpha^5\over\sqrt{5!}}|5\rangle+\dots\right) .

Read more about this topic:  Cat State

Famous quotes containing the word quantum:

    “The receipt to make a speaker, and an applauded one too, is short and easy.—Take of common sense quantum sufficit, add a little application to the rules and orders of the House, throw obvious thoughts in a new light, and make up the whole with a large quantity of purity, correctness, and elegancy of style.”
    —Philip Dormer Stanhope, 4th Earl Chesterfield (1694–1773)