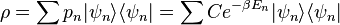Canonical Ensemble - Quantum Mechanical Systems

Quantum Mechanical Systems

By applying the canonical partition function, one can easily obtain the corresponding results for a canonical ensemble of quantum mechanical systems. A quantum mechanical ensemble in general is described by a density matrix. Suppose the Hamiltonian H of interest is a self adjoint operator with only discrete spectrum. The energy levels are then the eigenvalues of H, corresponding to eigenvector . From the same considerations as in the classical case, the probability that a system from the ensemble will be in state is, for some constant . So the ensemble is described by the density matrix

\rho = \sum p_n | \psi _n \rangle \langle \psi_n | = \sum C e^{- \beta E_n} | \psi _n \rangle \langle \psi_n|

(Technical note: a density matrix must be trace-class, therefore we have also assumed that the sequence of energy eigenvalues diverges sufficiently fast.) A density operator is assumed to have trace 1, so

which means

Q is the quantum-mechanical version of the canonical partition function. Putting C back into the equation for ρ gives

\rho = \frac{1}{\sum e^{- \beta E_n}} \sum e^{- \beta E_n} | \psi _n \rangle \langle \psi_n| = \frac{1}{ \operatorname{Tr}( e^{- \beta H} ) } e^{- \beta H} .

By the assumption that the energy eigenvalues diverge, the Hamiltonian H is an unbounded operator, therefore we have invoked the Borel functional calculus to exponentiate the Hamiltonian H. Alternatively, in non-rigorous fashion, one can consider that to be the exponential power series.

Notice the quantity

is the quantum mechanical counterpart of the canonical partition function, being the normalization factor for the mixed state of interest.

The density operator ρ obtained above therefore describes the (mixed) state of a canonical ensemble of quantum mechanical systems. As with any density operator, if A is a physical observable, then its expected value is

Read more about this topic:  Canonical Ensemble

Famous quotes containing the words quantum, mechanical and/or systems:

    “But how is one to make a scientist understand that there is something unalterably deranged about differential calculus, quantum theory, or the obscene and so inanely liturgical ordeals of the precession of the equinoxes.”
    —Antonin Artaud (1896–1948)

    “Industry has operated against the artisan in favor of the idler, and also in favor of capital and against labor. Any mechanical invention whatsoever has been more harmful to humanity than a century of war.”
    —Rémy De Gourmont (1858–1915)

    “We have done scant justice to the reasonableness of cannibalism. There are in fact so many and such excellent motives possible to it that mankind has never been able to fit all of them into one universal scheme, and has accordingly contrived various diverse and contradictory systems the better to display its virtues.”
    —Ruth Benedict (1887–1948)