Calibration Curve - Error in Calibration Curve Results

Error in Calibration Curve Results

As expected, the concentration of the unknown will have some error which can be calculated from the formula below. This formula assumes that a linear relationship is observed for all the standards. It is important to note that the error in the concentration will be minimal if the signal from the unknown lies in the middle of the signals of all the standards (the term goes to zero if )

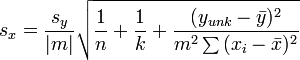
s_x=\frac{s_y}{|m|}\sqrt{\frac{1}{n}+\frac{1}{k}+\frac{(y_{unk}-\bar{y})^2}{m^2\sum{(x_i-\bar{x})^2}}}

  • is the standard deviation in the residuals
  • is the slope of the line
  • is the y-intercept of the line
  • is the number standards
  • is the number of replicate unknowns
  • is the measurement of the unknown
  • is the average measurement of the standards
  • are the concentrations of the standards
  • is the average concentration of the standards

Read more about this topic:  Calibration Curve

Famous quotes containing the words error in, error, curve and/or results:

    “‘Tis this desire of bending all things to our own purposes which turns them into confusion and is the chief source of every error in our lives.”
    —Sarah Fielding (1710–1768)

    “An error is the more dangerous in proportion to the degree of truth which it contains.”
    —Henri-Frédéric Amiel (1821–1881)

    “The years-heired feature that can
    In curve and voice and eye
    Despise the human span
    Of durance—that is I;
    The eternal thing in man,
    That heeds no call to die.”
    —Thomas Hardy (1840–1928)

    “Pain itself can be pleasurable accidentally in so far as it is accompanied by wonder, as in stage-plays; or in so far as it recalls a beloved object to one’s memory, and makes one feel one’s love for the thing, whose absence gives us pain. Consequently, since love is pleasant, both pain and whatever else results from love, in so far as they remind us of our love, are pleasant.”
    —Thomas Aquinas (c. 1225–1274)