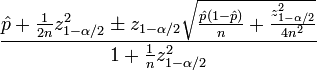Binomial Proportion Confidence Interval - Wilson Score Interval

Wilson Score Interval

The Wilson interval is an improvement (the actual coverage probability is closer to the nominal value) over the normal approximation interval and was first developed by Edwin Bidwell Wilson (1927).

\frac{{ {\hat p + \frac{{1}}{{2n}} z_{1- \alpha / 2}^2 \pm z_{1- \alpha / 2} \sqrt {\frac{{\hat p\left( {1 - \hat p} \right)}}{n} + \frac{{z_{1- \alpha / 2}^2}} {{4n^2}} }} }} {{ {1 + \frac{{1}}{n}} z_{1- \alpha / 2}^2 }}

This interval has good properties even for a small number of trials and/or an extreme probability. The center of the Wilson interval

\frac{{ {\hat p + \frac{{1}}{{2n}} z_{1- \alpha / 2}^2 } }} {{ {1 + \frac{{1}}{n}} z_{1- \alpha / 2}^2 }}

can be shown to be a weighted average of and, with receiving greater weight as the sample size increases. For the 95% interval, the Wilson interval is nearly identical to the normal approximation interval using instead of .

The Wilson interval can be derived from Pearson's chi-squared test with two categories. The resulting interval

can then be solved for to produce the Wilson interval.

The test in the middle of the inequality is a score test, so the Wilson interval is sometimes called the Wilson score interval.

Read more about this topic:  Binomial Proportion Confidence Interval

Famous quotes containing the words wilson, score and/or interval:

    “The spirit of [William] Penn will not be stayed. You cannot set limits to such knightly adventurers. After their own day is gone their spirits stalk the world, carrying inspiration everywhere that they go and reminding men of the lineage, the fine lineage, of those who have sought justice and right.”
    —Woodrow Wilson (1856–1924)

    “Earthly minds, like mud walls, resist the strongest batteries: and though, perhaps, sometimes the force of a clear argument may make some impression, yet they nevertheless stand firm, and keep out the enemy, truth, that would captivate or disturb them. Tell a man passionately in love, that he is jilted; bring a score of witnesses of the falsehood of his mistress, it is ten to one but three kind words of hers shall invalidate all their testimonies.”
    —John Locke (1632–1704)

    “[I have] been in love with one princess or another almost all my life, and I hope I shall go on so, till I die, being firmly persuaded, that if ever I do a mean action, it must be in some interval betwixt one passion and another.”
    —Laurence Sterne (1713–1768)