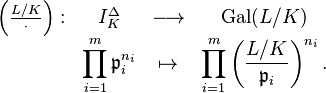Artin Reciprocity Law - Finite Extensions of Global Fields

Finite Extensions of Global Fields

The definition of the Artin map for a finite abelian extension L/K of global fields (such as a finite abelian extension of Q) has a concrete description in terms of prime ideals and Frobenius elements.

If is a prime of K then the decomposition groups of primes above are equal in Gal(L/K) since the latter group is abelian. If is unramified in L, then the decomposition group is canonically isomorphic to the Galois group of the extension of residue fields over . There is therefore a canonically defined Frobenius element in Gal(L/K) denoted by or . If Δ denotes the relative discriminant of L/K, the Artin symbol (or Artin map, or (global) reciprocity map) of L/K is defined on the group of prime-to-Δ fractional ideals, by linearity:

\begin{matrix} \left(\frac{L/K}{\cdot}\right):&I_K^\Delta&\longrightarrow&\mathrm{Gal}(L/K)\\ &\displaystyle{\prod_{i=1}^m\mathfrak{p}_i^{n_i}}&\mapsto&\displaystyle{\prod_{i=1}^m\left(\frac{L/K}{\mathfrak{p}_i}\right)^{n_i}.} \end{matrix}

The Artin reciprocity law (or global reciprocity law) states that there is a modulus c of K such that the Artin map induces an isomorphism

where Kc,1 is the ray modulo c, NmL/K is the norm map associated to L/K and is the fractional ideals of L prime to c. Such a modulus c is called a defining modulus for L/K. The smallest defining modulus is called the conductor of L/K and typically denoted .

Read more about this topic:  Artin Reciprocity Law

Famous quotes containing the words finite, extensions, global and/or fields:

    “All finite things reveal infinitude:”
    —Theodore Roethke (1908–1963)

    “If we focus exclusively on teaching our children to read, write, spell, and count in their first years of life, we turn our homes into extensions of school and turn bringing up a child into an exercise in curriculum development. We should be parents first and teachers of academic skills second.”
    —Neil Kurshan (20th century)

    “The Sage of Toronto ... spent several decades marveling at the numerous freedoms created by a “global village” instantly and effortlessly accessible to all. Villages, unlike towns, have always been ruled by conformism, isolation, petty surveillance, boredom and repetitive malicious gossip about the same families. Which is a precise enough description of the global spectacle’s present vulgarity.”
    —Guy Debord (b. 1931)

    “Within the regions of the air,
    Compassed about with heavens fair,
    Great tracts of land there may be found
    Enriched with fields and fertile ground;
    Where many numerous hosts
    In those far distant coasts,
    For other great and glorious ends,
    Inhabit, my yet unknown friends.”
    —Thomas Traherne (1636–1674)