Theoretical Aspects of Far-field/near-field Balance
The drag force calculation can be performed using the integral of force balance in the freestream direction as
which surrounds the body represents the union of two unconnected surfaces,
where is the airplane surface, is the outlet surface and represents both the lateral and inlet surfaces. In general, the far-field control volume is located in the boundaries of the domain and its choice is user-defined. In Subsection \ref{sGF}, further considerations concerning to the correct selection of the far-field boundary are given, allowing for desired flow characteristics.
Equation (\ref{ta1}) can be decomposed into two surface integrals, yielding
The right-hand side integral in Eq.\ (\ref{ta2}) represents the reaction forces of the airplane. The left-hand side integral in Eq.\ (\ref{ta2}) represents the total force exerted by the fluid. Mathematically, these two integrals are equivalent. However, the numerical integration of these terms will hardly lead to the same result, because the solution is approximated. In the terminology of Computational Fluid Dynamics (CFD), when the integration is performed using the left-hand side integral in Eq.\ (\ref{ta2}), the near-field method is employed. On the other hand, when the integration of the right-hand side in Eq. (\ref{ta2}) is computed, the far-field method is considered.
The drag force balance is assured mathematically by Eq.\ (\ref{ta2}), that is, the resultant drag force evaluated using the near-field approach must be equal to the drag force extracted by the far-field approach. The correct drag breakdown considered in this work is
Read more about this topic: Aerodynamic Drag
Famous quotes containing the words theoretical, aspects and/or balance:
“The desire to serve the common good must without fail be a requisite of the soul, a necessity for personal happiness; if it issues not from there, but from theoretical or other considerations, it is not at all the same thing.”
—Anton Pavlovich Chekhov (1860–1904)
“The North American system only wants to consider the positive aspects of reality. Men and women are subjected from childhood to an inexorable process of adaptation; certain principles, contained in brief formulas are endlessly repeated by the press, the radio, the churches, and the schools, and by those kindly, sinister beings, the North American mothers and wives. A person imprisoned by these schemes is like a plant in a flowerpot too small for it: he cannot grow or mature.”
—Octavio Paz (b. 1914)
“Genius has infused itself into nature. It indicates itself by a small excess of good, a small balance in brute facts always favorable to the side of reason.”
—Ralph Waldo Emerson (1803–1882)
![\int_{S=S_{\infty}+S_D+S_A}\left[\rho\,u\,\vec{q}+\left(p-p_{\infty}\right)
\vec{i} - \vec{\tau}_{x}\right].\vec{n}dS\,=\,0](http://upload.wikimedia.org/math/e/8/f/e8f22bbc6a88988aae675dce592efa11.png)
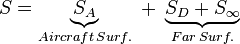
![\int_{S_{A}}\left[\rho\,u\,\vec{q}+\left(p-p_{\infty}\right)
\vec{i} -\vec{\tau}_{x}\right]\,.\,\vec{n}\,dS =-\int_{S_{D}+S_{\infty}}\left[\rho\,u\,\vec{q}+\left(p-p_{\infty}\right)
\vec{i} -\vec{\tau}_{x}\right]\,.\,\vec{n}\,dS](http://upload.wikimedia.org/math/3/d/d/3dd4d96b9e0d818dde349bf339d80902.png)
