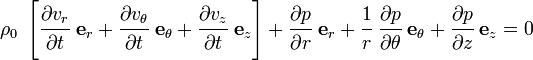Acoustic Theory - Governing Equations in Cylindrical Coordinates

Governing Equations in Cylindrical Coordinates

If we use a cylindrical coordinate system with basis vectors, then the gradient of and the divergence of are given by

\begin{align} \nabla p & = \cfrac{\partial p}{\partial r}~\mathbf{e}_r + \cfrac{1}{r}~\cfrac{\partial p}{\partial \theta}~\mathbf{e}_\theta + \cfrac{\partial p}{\partial z}~\mathbf{e}_z \\ \nabla\cdot\mathbf{v} & = \cfrac{\partial v_r}{\partial r} + \cfrac{1}{r}\left(\cfrac{\partial v_\theta}{\partial \theta} + v_r\right) + \cfrac{\partial v_z}{\partial z} \end{align}

where the velocity has been expressed as .

The equations for the conservation of momentum may then be written as

\rho_0~\left + \cfrac{\partial p}{\partial r}~\mathbf{e}_r + \cfrac{1}{r}~\cfrac{\partial p}{\partial \theta}~\mathbf{e}_\theta + \cfrac{\partial p}{\partial z}~\mathbf{e}_z = 0

In terms of components, these three equations for the conservation of momentum in cylindrical coordinates are

\rho_0~\cfrac{\partial v_r}{\partial t} + \cfrac{\partial p}{\partial r} = 0 ~;~~ \rho_0~\cfrac{\partial v_\theta}{\partial t} + \cfrac{1}{r}~\cfrac{\partial p}{\partial \theta} = 0 ~;~~ \rho_0~\cfrac{\partial v_z}{\partial t} + \cfrac{\partial p}{\partial z} = 0 ~.

The equation for the conservation of mass can similarly be written in cylindrical coordinates as

\cfrac{\partial p}{\partial t} + \kappa\left = 0 ~.

Read more about this topic:  Acoustic Theory

Famous quotes containing the word governing:

    “We are apt to say that a foreign policy is successful only when the country, or at any rate the governing class, is united behind it. In reality, every line of policy is repudiated by a section, often by an influential section, of the country concerned. A foreign minister who waited until everyone agreed with him would have no foreign policy at all.”
    —A.J.P. (Alan John Percivale)